Data Update 2 for 2021: The Price of Risk!
Investors are constantly in search of a single metric that will tell them whether a market is under or over valued, and consequently whether they should buying or selling holdings in that market. With equities, the metric that has been in use the longest is the PE ratio, modified in recent years to the CAPE, where earnings are normalized (by averaging over time) and sometimes adjusted for inflation. That metric, though, has been signaling that stocks are over valued for most of the last decade, a ten-year period when stocks delivered blockbuster returns. The failures of the signal have been variously attributed to low interest rates, accounting mis-measurement of earnings (especially at tech companies), and by some, to animal spirits. In this post, I offer an alternative, albeit a more complicated, metric that I believe offers not only a more comprehensive measure of pricing, but also operates as a barometer of the ups and downs in the market.
The Price of Risk
The price of risk is what investors demand as a premium, an extra return over and above what they can make on a guaranteed investment (risk free), to invest in a risky asset. Note that this price is set by demand and supply and will reflect everything that investors collectively believe, hope for, and fear.

Does the price of risk have to be positive? The answer depends on whether human beings are risk averse or not. If they are, the price of risk will be reflected in a positive premium, and the level of the premium will increase, as investors become more risk averse. If, on the other hand, investors are risk neutral, the price of risk will be zero, and investors will buy risky business, stocks and other investments, and settle for the risk free rate as the expected return.
Note that nothing that I have said so far is premised on modern portfolio theory, or any academic view of risk premiums. It is true that economists have researched risk aversion for centuries and concluded that investors are collectively risk averse, and that the level of risk aversion varies across age groups, income levels and time. Some have developed models that try to measure what a fair risk premium should be, but to arrive at their conclusions, they have make assumptions about investor utility functions that are often unobservable and untestable. I have no desire to make this a lengthy treatise about the "right" risk premium, but will instead start with two assertions:
Risk premiums can be estimated: You can back out the risk premiums that investors are demanding from the prices that they pay for risky assets. Put simply, if you can observe the price that an investor pays for a risky asset, and are willing to estimate the expected cash flows on that asset, you can estimate the expected return on that asset and net out the risk free asset to arrive at a risk premium. It is true that you can make mistakes on your expected cash flows, but your output should reflect an estimate, albeit a noisy one, of what investors are demanding as a premium.
Risk premiums can and will change over time: Risk premiums are driven by risk aversion, and risk aversion itself can change over time. In fact, greed and fear, two big drivers of market prices, also affect risk aversion, with investors becoming more risk averse and charging higher premiums, when the fear factor becomes dominant.
When risk premiums change, prices will move: As risk premiums change, the prices that investors are willing to pay for risky assets will also change, with the two moving in opposite directions. Intuitively, if you want to earn a higher risk premium on an investment, holding cash flows fixed, you will pay less for that investment today.
The Price of Risk: Bond Market
All bonds, including those with guaranteed coupons, are risky, if you define risk as prices being volatile, since as interest rates changes, bond prices will change as well. Most bonds, though, are exposed to a second risk, which is that the bond issuer can default on coupon payments, making returns and prices even more uncertain. This is why corporate bonds are riskier than sovereign bonds, and sovereign bonds issued by shakier governments are riskier than sovereign bonds issued by governments that are unlikely to default.
Bond Default Spread
If you accept the proposition that a bond with default risk is riskier than an otherwise equivalent bond (same coupon and maturity) issued by a default-free entity, the price of risk in the bond market can be measured by looking at the differences in yields between the two bonds. Thus, if a 10-year corporate bond has a yield of 3.00% and a 10-year government bond, in the same currency and with no default risk, has a yield of 1.00%, the difference is termed the default spread and becomes a measure of the price of risk in the bond market.
At the risk of belaboring the details, it is not the yield that we should be comparing, but the yield to maturity, which is the internal rate of return on the bond, given how it is priced:
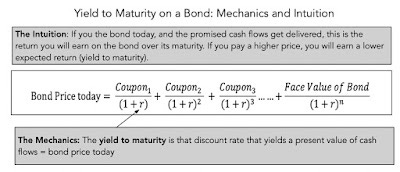
To compute the default spread over a 10-year period for a specific corporate bond (or loan), you would compute the yields to maturity on the ten-year corporate and treasury bonds and take the difference. Note that even this comparison is an approximation, but it yields a close enough value to work, and that it yields a default spread for a specific maturity. You could compute default spreads for other maturities, and compute the price of risk over 1-year, 2-year, 3-year periods and so on.
Corporate Default Spreads: Current and Look Back at 2020
Corporate bonds are traded, and as a consequence, and you can use traded prices to estimate default spreads in the market. In the chart below, I compare default spreads at the start of 2021 with the default spreads at the start of 2020:
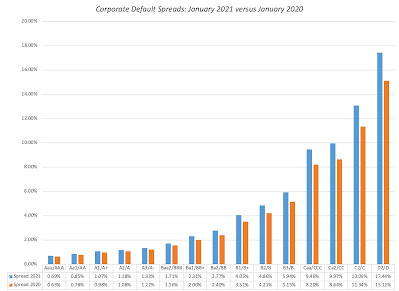
Source: BofA ML Spreads on Federal Reserve (FRED)
At first sight, it looks like an uneventful year, with spreads in 2021 mildly higher than spreads in 2020, but that comparison is deceptive, since default spreads went on a roller-coaster ride during 2020:
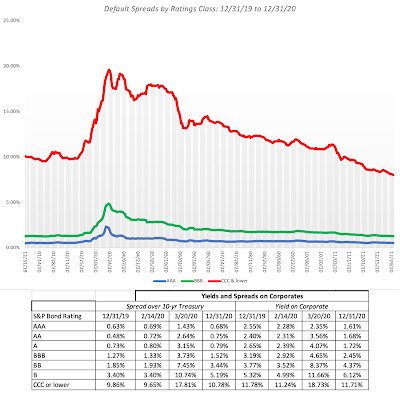
Source: BofA ML Spreads on Federal Reserve (FRED)
While spreads started 2020 in serene fashion, the COVID-driven market crisis caused them to widen dramatically between February 14 and March 20, with the spreads almost tripling for lower rated bonds. Given the worries about default and a full-fledged market meltdown, that was not surprising, but what is surprising is how quickly the fear factor faded and spreads returned almost to pre-crisis levels.
Measuring against the past
Are default spreads today too low? There are two ways to answer that question. One is to look at their movement over time, and compare current spreads to historic norms.
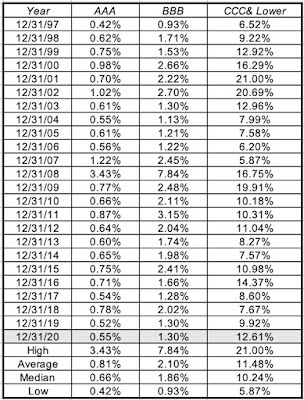
Source: BofA ML Spreads on Federal Reserve (FRED)
The default spreads at the end of 2020 are at the low end of the historical spectrum, and the contrast with the 2008 crisis is stark, since default spread surged in the last quarter of 2008 and did not come back down to pre-crisis levels until almost two years later. The other is to look at corporate defaults over time to see if markets are building in enough of a buffer against future defaults.
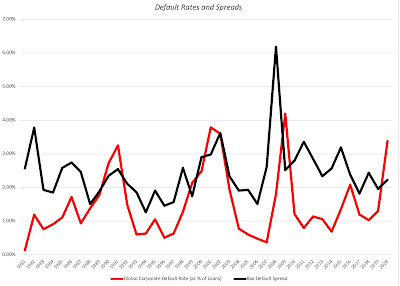
Sources: S&P and Moody's
Default rates increased in 2020, with spillover effects expected into 2021, but the corporate bond default spreads do not seem to reflect this. One explanation is that the bond market beliefs that the worst of the crisis is over and that default rates will return quickly to pre-COVID levels. The other is the corporate bond market is under estimating both the risk and the consequences of default.
The Price of Risk: Equities
Equities are riskier than bonds (or at least most bonds), and it stands to reason that there is a price of risk bearing in the equity markets. While that price has a name, i.e., the equity risk premium, it is more difficult to observe and estimate than the default spread in bond markets. In this section, I will present both the standard approach to estimating the equity risk premium and my preferred way of doing so, with a rationale for why.
Estimation Approaches
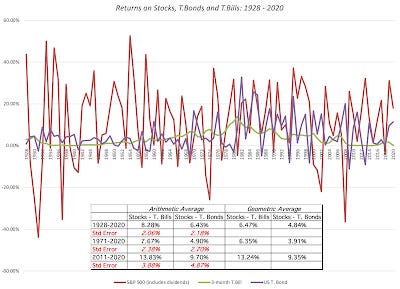
Why is it so difficult to estimate an equity risk premium? The simple reason is that unlike a bond, which comes with specified coupons, the cash flows that you receive when you buy stocks are neither pre-specified nor guaranteed. It is true that some companies pay dividends, and that these dividends are sticky, but it is also true that companies are under no contractual obligation to continue paying those same dividends. This difficulty in observing the equity risk premium leads many to look backwards, when asked to estimate the equity risk premium. Put simply they look at a long time period in the past (50 years or even 100 years) and look at the premium that stocks earned over a risk free investment (treasury bills or bonds); that historical risk premium then gets used as a measure of the current equity risk premium. On my website, I update this historical risk premium every year, and the graph below reflects my January 2021 findings:
Download spreadsheet with raw data
Looking over a 92-year time period (1928-2020), for instance, stocks earned an geometric average return of 9.79%, giving them a premium of 4.84% over the 4.95% that you would have earned, investing in treasury bonds. If you buy into this measure of equity risk premiums, consider its limitations. First, it is backward looking and built on the presumption that the future will look like the past. Second, even if you trust mean reversion, note that the estimated premium is not a fact but an estimate, with a wide range around it. Specifically, the estimate of 4.84% for the equity risk premium from 1928 to 2020 comes with a standard error of 2.1%; the true ERP, with this error, could fall anywhere from 0.64% to 9.04%. Third, this premium is static and does not reflect market crises and investor fears; thus, the historical risk premium on February 14, 2020 would have very similar to the historical risk premium on March 20, 2020.
The alternative approach to estimating equity risk premiums is revolutionary and it borrows from the yield to maturity approach that we used to estimate bond default spreads. Consider replacing the bond price with the level of stock prices today (say, with the S&P 500 index) and coupons with expected cash flows on stocks (from dividends and buybacks), and solve for an internal rate of return:
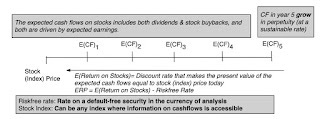
Implied Equity Risk Premium: In General
The internal rate of return is the expected return on stocks, and netting out the risk free rate today will yield an implied equity risk premium. In the picture below, I use this process to estimate an equity risk premium of 4.72% for the S&P 500 on January 1, 2021:
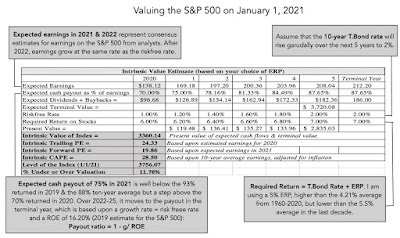
Download spreadsheet to compute ERP
It is true that my estimates of earnings and cash flows in the future are driving my premium, and that the premium will be lower (higher) if I have under (over) estimated those numbers. This approach to estimating equity risk premiums is forward-looking and dynamic, changing as the market price changes. In the graph below, I report implied equity risk premiums that I computed, by day, during 2020, in an effort to gauge how the crisis was playing out and keep my sanity.
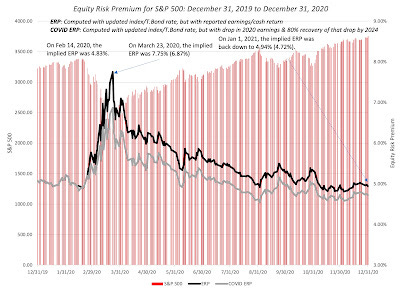
Download spreadsheet with data
As with the bond default spread, the implied equity risk premium was extraordinarily volatile in 2020, peaking at 7.75% on March 20, before falling back to pre-crisis levels by the end of the year.
Market Gauge?
As we are engulfed by talk of market bubbles and corrections, it is worth nothing that any question about the overall market can really be reframed as a question about the implied equity risk premium. If you believe that the current implied equity risk premium is too low, you are in effect also saying that stocks are overvalued, just as a judgment that the equity risk premium is too high is equivalent to arguing that stocks are undervalued. So, at 4.72%, is the equity risk premium too low and is the market in a bubble? One way to pass judgment is to compare the current premium to implied equity risk premiums in the past:
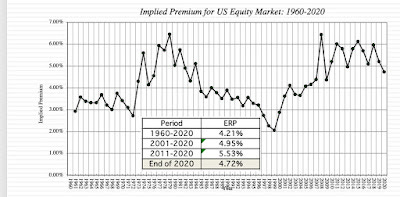
Download spreadsheet with historical ERP
On this comparison, stocks don't look significantly over valued, since the current premium is higher than the long term average (4.21%), though if you compare it to the equity risk premium in the last decade (5.53%), it looks low, and that stocks are over valued by about 15%. There is a caveat, though, which is that this risk premium is being earned on a risk free rate that is historically low. Consider this alternative graph, where I look at the expected return on stocks (risk free rate plus implied equity risk premium) over the same time period:
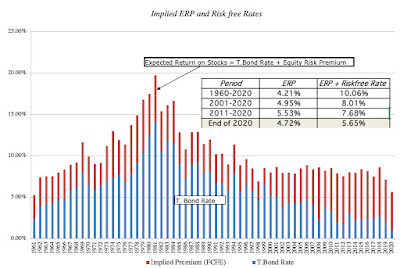
For much of this century, the expected return on stocks has hovered around 8%, but the expected return at the start of 2021 is only 5.65%, well below the expected returns in prior periods.
A Market Assessment
I know that you are probably incredibly confused, and I am afraid that I cannot clear up all of that confusion, but this framework lends itself to valuing the entire market. To do this, you have to be willing to make estimates of:
Earnings on the index: You cannot value a market based upon last year's earnings (though many do so). Investing is about the future, and uncomfortable as it may make you feel, you have to make estimates for the future. With an index like the S&P 500, you can outsource these estimates at least for the near years, by looking at consensus forecasts from analysts tracking the index.
Cash returned, relative to earnings: Since it is cash returned to stockholders that drives value, you also have to make judgments on what percent of earnings will be returned to stockholders, either in dividends or buybacks. To this, you can look to history, but recognize that it is also a function of the confidence that companies have about the future, with more confidence leading to higher cash being returned.
Risk free rates over time: While it is generally not a good idea to play interest rate forecaster, we are in unusual times, with rates close to all time lows. In addition, your views on future growth in the economy are intertwined with what will happen to risk free rates, with stronger economic growth putting more upward pressure on rates.
An acceptable ERP: As I noted in the last section, equity risk premiums have been volatile over time, and particularly so in years in 2020. The equity risk premium, added to the risk free rate, will determine what you need stock returns to be, to break even on a risk-adjusted basis.
It is only fair that I go first. In the picture below, I make my best judgments on each of these dimensions, using consensus estimates of earnings in 2021 and 2022 to get started, but then slowing growth in earnings to match the growth rate in the economy, which I approximate with the risk free rate. On the risk free rate, I assume that rates will rise over time to 2%, and that 5% is a fair ERP, given history. My valuation is below:
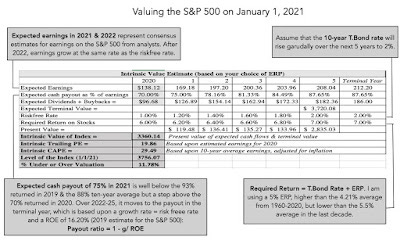
Download spreadsheet to value S&P 500
Based upon my inputs, the S&P 500 is over valued by about 12%, certainly not bubble territory, but still richly priced. You may (and should) disagree with my assumptions, and I welcome you to download the spreadsheet and change the inputs. Ultimately though, the judgment you make on the market will be a joint effect of your views on the economy and interest rates in the next few years. The table below summarizes the interplay between economic growth and interest rate assumptions, and the effects on the index value:
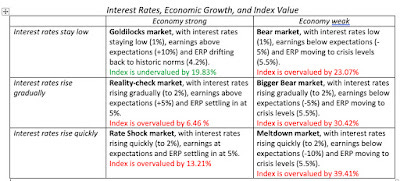
Use S&P 500 valuation spreadsheet to assess value effects
As you can see, there are far more bad possible outcomes than good ones, and the only scenario where stocks have significant room to rise is the Goldilocks market, where rates stay low (at close to 1%), while the economy comes back strongly. I know that the possibility of additional economic stimulus may improve the odds for the economy, but can they do so without affecting rates? To buy into that scenario, you have to belief that the Fed has the power to keep rates low, no matter what happens to the economy, and I don't share that faith.
As many of you who have read my blog posts know, I am a reluctant market timer, but ultimately we all time markets, implicitly or explicitly, the former wooing up in how much of your portfolio is in cash and the latter in more overt acts of either protection or bets on market directions. Going into 2021, I have far more cash in my portfolio than I usually do, and for the first time in a long, long time, I have bought partial protection against a market drop, using derivatives. It is insurance, and like all insurance, my best case scenario is that I never need to use it, but it reflects my wariness about what comes next. I am not and don't want to be in the business of doling out investment advice, and I think that the healthiest pathway for you is to make your own judgments on interest rates, earnings growth and acceptable risk premiums, and follow that with consistent actions.
YouTube Video
Spreadsheets
Data Updates for 2021